GRE Questions

---

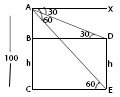
From above diagram
AC represents the hill and DE represents the tower

Given that AC = 100 m

angleXAD = angleADB = 30° (∵ AX || BD )
angleXAE = angleAEC = 60° (∵ AX || CE)

Let DE = h

Then, BC = DE = h, AB = (100-h) (∵ AC=100 and BC = h), BD = CE

tan 60°=AC/CE => √3 = 100/CE =>CE = 100/√3 ----- (1)

tan 30° = AB/BD => 1/√3 = 100−h/BD => BD = 100−h(√3)
∵ BD = CE and Substitute the value of CE from equation 1
100/√3 = 100−h(√3) => h = 66.66 mts

The height of the tower = 66.66 mts.

The first person shakes hands with 22 different people, the second person also shakes hands with 22 different people, but one of those handshakes was counted in the 22 for the first person, so the second person actually shakes hands with 21 new people. The third person, 20 people, and so on...
So,
22 + 21 + 20 + 19 + 18 + 17 + 16 + 15 + 14 + 13 + 12 + 11 + 10 + 9 + 8 + 7 + 6 + 5 + 4 + 3 + 2 + 1
= n(n+1)/2 = 22 x 23 /2 = 11 x 23 = 253.

Average speed=total distance/total time
Let distance to store be  K
then, total time =(K/20)+(K/30)=K/12
and, total time =(2K)
so average speed= 2K / (K/12) = 24kmph.

Donkeys and horses can be breed to make mules and hinnys.

A hinny is a domestic equine hybrid that is the offspring of a male horse, a stallion, and a female donkey, a jenny. It is the reciprocal cross to the more common mule, which is the product of a male donkey, a jack, and a female horse, a mare.

It is wrong for doctors to lie about their patients' ...........illnesses. From the above statements this will be true

Given share of L is 2/7 th of profit. Then, their profits are divided in to 5:2 ratio.

Ratio of K and L is

16000×8x×4=52

x = Rs. 12,800.

Putting A=1, B=2, C=3,..., M=13,....,X=24,Y=25, Z=26, we have :

 JR = J + R2 + 2 = 10 + 18 2 + 2 = 16

CK = C + K2 + 2 = 3 + 112 + 2 = 9

TX = T + X2+ 2 = 20 + 242 + 2 = 24

OS = O + S2 + 2 = 15 + 192 + 2 = 19

 So, the missing numbers are :

21 and 14

Hence, the answer is (b).